Compulsory courses, M2 First semester, courses
Stochastic processes
Stochastic phenomena, the description of which involves randomness, are ubiquitous in physics, chemistry, biology and beyond (information theory, computer science, economy etc.). The goal is to equip students with the essential tools for their modeling and understanding. The course starts with a probability bootstrap with emphasis on large deviations, establishing a bridge with statistical physics approaches. Markov processes, Langevin and Fokker-Planck equations are then presented, together with the stochastic differential equation framework and stochastic calculus (Stratonovich versus Ito-Döblin rules). The linear-response theory features, found along the way, will be put in a broader perspective. The second half of the course is devoted to fluctuation theorems, first passage properties. Follows an introduction to functionals of Brownian motion and the Feynman-Kac formula.
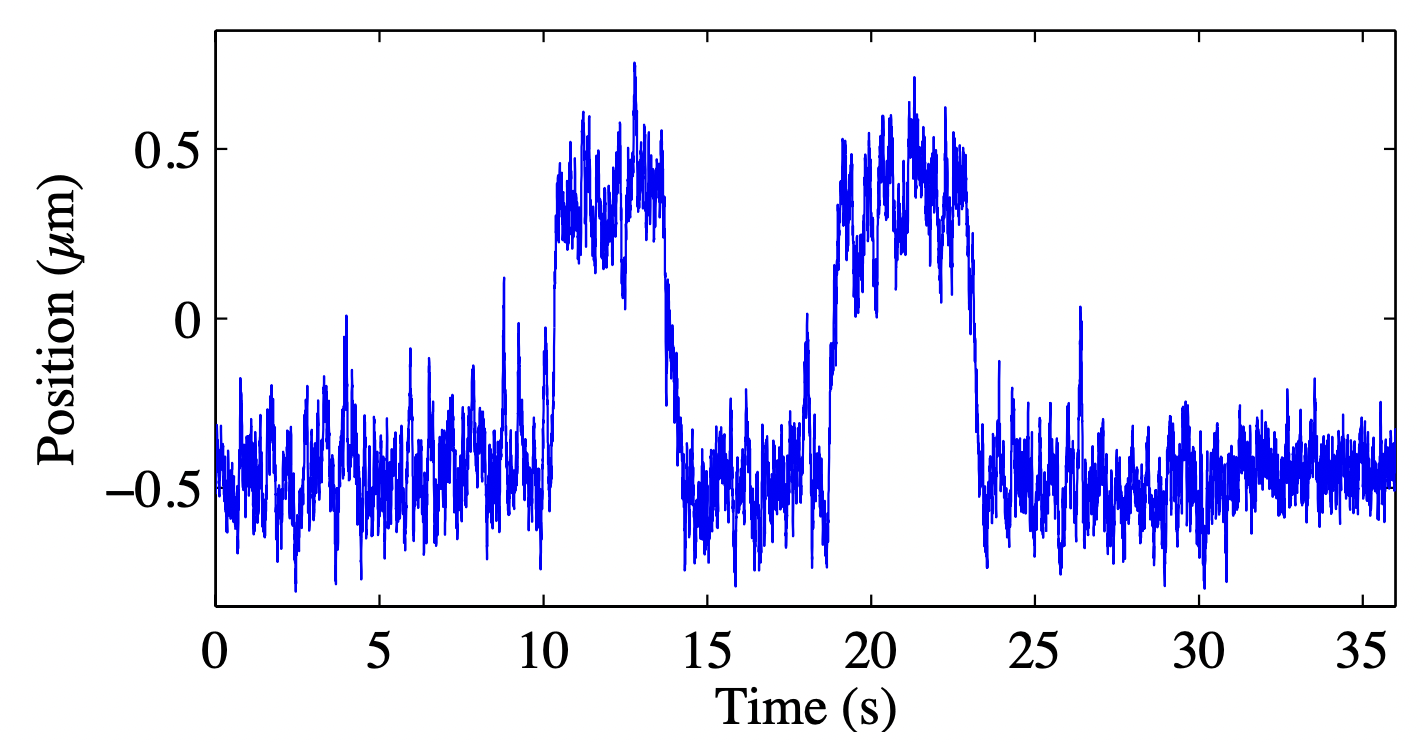
Trajectory of a colloidal particle trapped in a modulated double-well potential ; from A. Berut et al., J. Stat. Mech P06015 (2015)
Few references:
- The Fokker-Planck Equation: Methods of Solutions and Applications, H. Risken, Springer.
- Stochastic processes in physics and chemistry, N. G. van Kampen, Elsevier.
- Handbook of stochastic methods, C. W. Gardiner, Springer.
 Christophe Texier (Université Paris-Saclay)
Christophe Texier (Université Paris-Saclay)
Comments are closed





